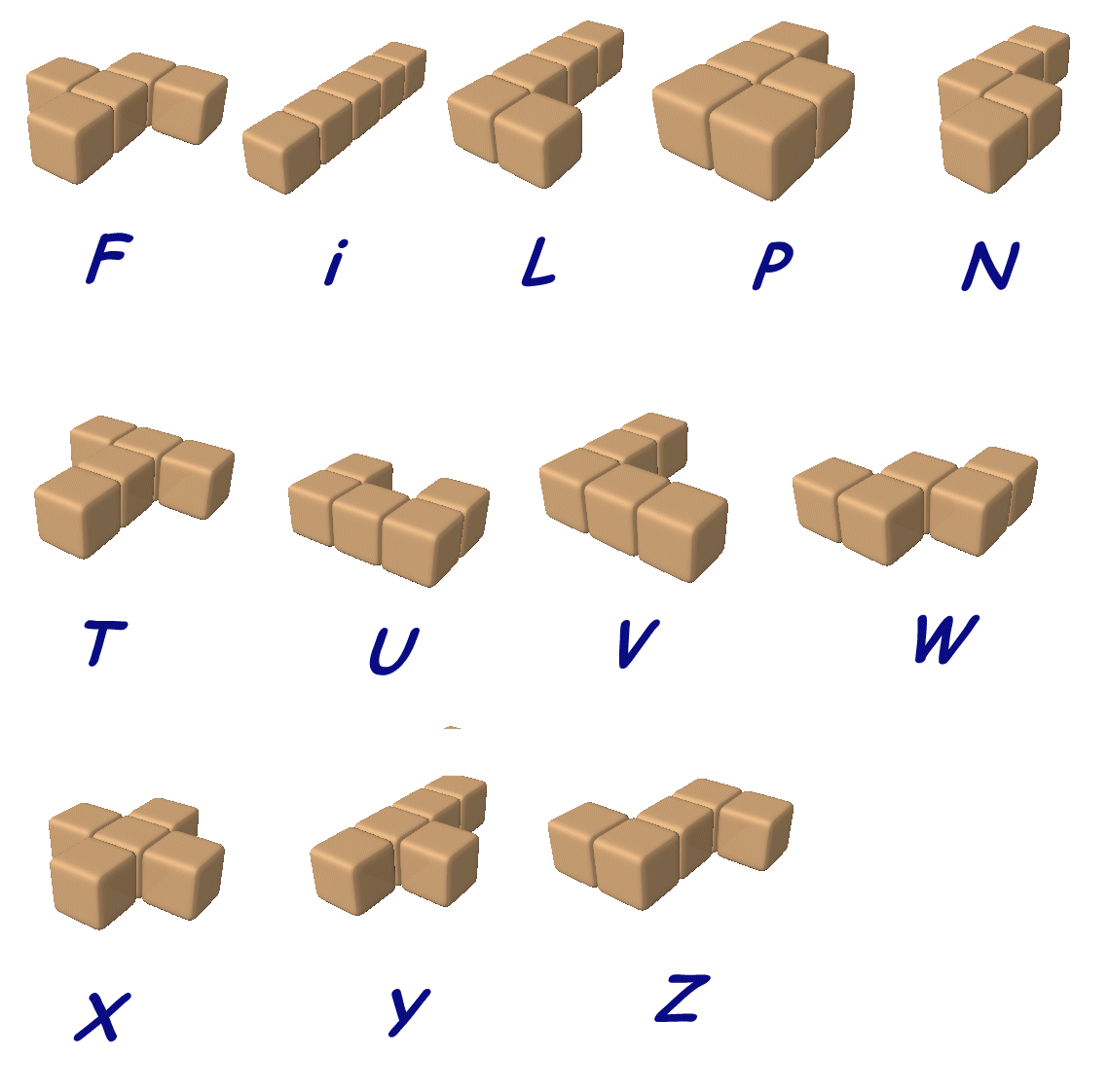GUIDE TO PENTOMINOES

The Pentominoes Concept can be traced to the ancient Chinese game of 'Go' (played by placing black and white stone markers upon a board with a special grid marked upon it). The aim of that game is to surround your opponent's stones with your own stones and as part of his strategic deliberations upon strategy in this game, an ancient 'Go' master noted that twelve distinctive patterns can be formed by five connected stones on the board. This is the core concept of a set of Pentominoes, although this ancient master had not given them their name at that time.

The first Pentominoes problem was published in The Canterbury Puzzles in 1907 by Henry Ernest Dudney but they were not given their present name or presented to the general public until Solomon W. Golomb gave a talk to the Harvard Mathematics club in 1953 which was then partly published in Scientific American in 1957.

He referred to 'Shapes that use five square blocks joined together with at least one common side' and named them 'Pentominoes'.

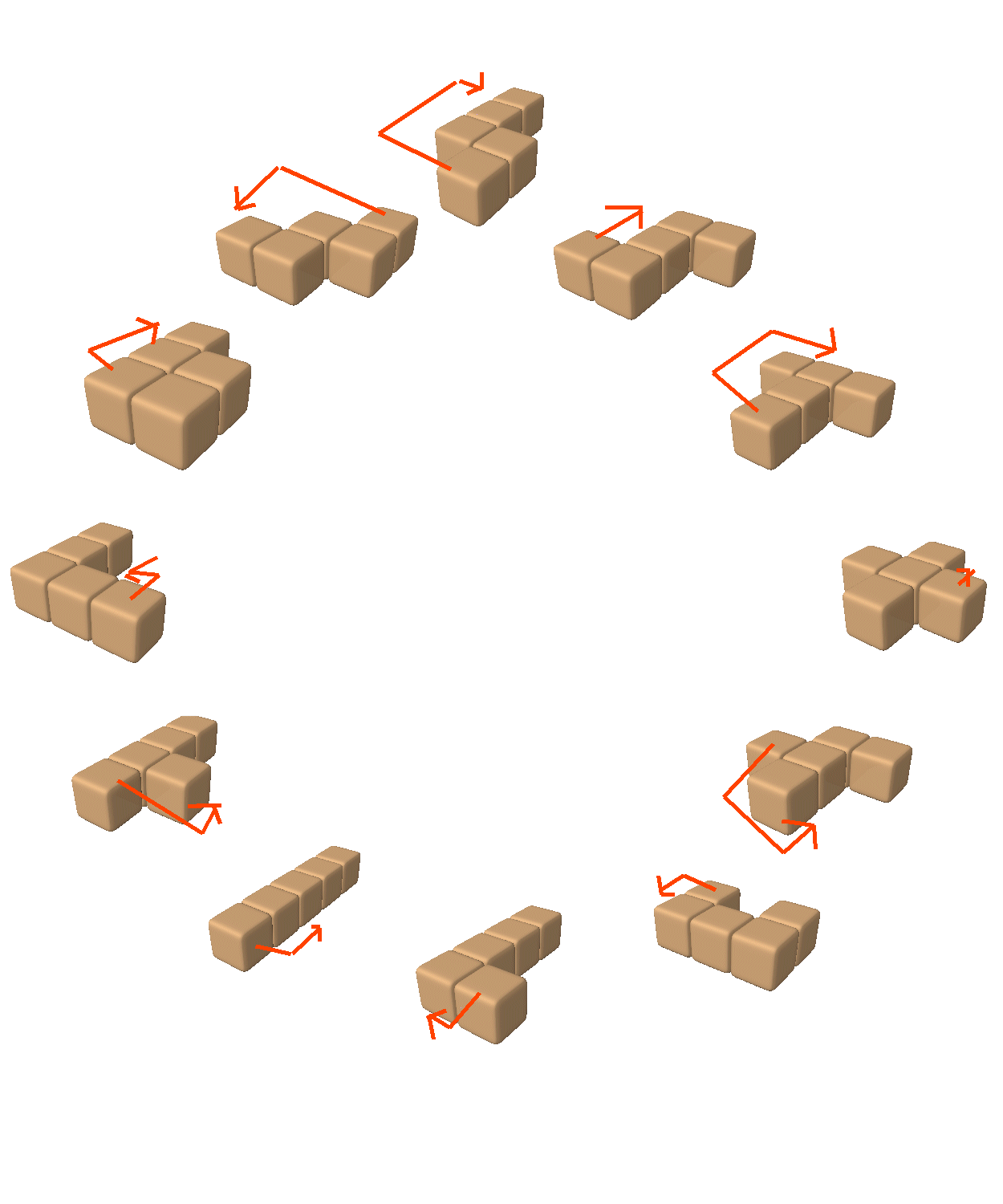
You can work out the 12 shapes systematically by moving one square at a time until you reach the shape you started with, if you follow the following sequence:

The 12 shapes are now affectionately referred to by devotees using their letter names and you can use these to remind yourself of the full set of shapes by recounting the letters of the alphabet after and including the letter 'T' plus selected letters from the peculiarly spelled word: FILiPiNo.

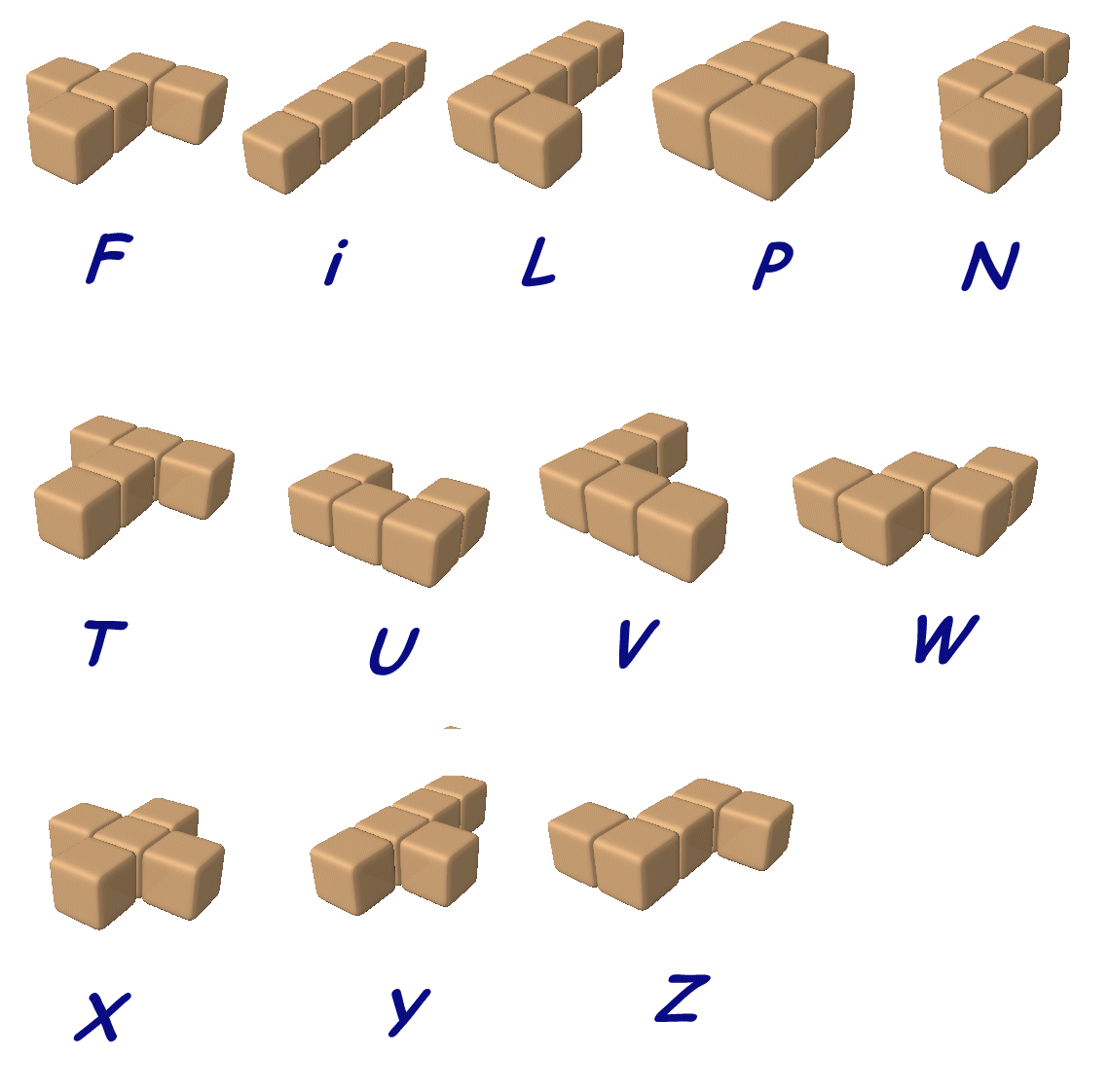
The 'magic' of this set of shapes is to be found in the large variety of 'puzzles' that you can set yourself when working with them. Working on the puzzles (particularly those in 3 dimensions) has been shown to greatly improve spatial awareness and perception, and of course there is great satisfaction to be found in working your way through to a solution without looking it up!

The rectangular 2-dimensional puzzles are identified by their dimensions: 6 x 10, 5 x 12, 4 x 15, and 3 x 20 rectangles using all twelve pentominoes. The 3 x 20 is the most difficult to derive, and there is only one unique solution, except for the possibility of rotating the shaded central portion by 180 degrees.

There are a wide variety of other shapes, including:

There are also a wide variety of 3 dimensional shapes:

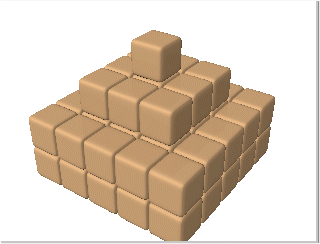
Since there are twelve distinct pentomino shapes with each covering five squares, their total area is sixty squares. There are several ways to place all twelve different pentominoes on an 8 x 8 board, with four squares always left over. By artistically specifying the positions of the four extra squares, many interesting patterns can be created. Another evident possibility is to require that the four extra squares form a 2 x 2 area (a square tetromino) in a specified position on the board. This placement leads to a remarkable theorem, which can be proved by using only three constructions: Wherever on the checkerboard a square tetromino is placed, the rest of the board can be covered with the twelve pentominoes.

The 8x8 puzzles:

Another problem is to determine the least number of pentominoes which will span the checkerboard. In other words, some of the pentominoes are placed on the board in such a way that none of the rest can be added. Although there are several different configurations for solving this puzzle, the minimum number is five pentominoes.

R. M. Robinson, professor of mathematics at the University of California at Berkley, has suggested another intriguing construction with pentominoes, which he calls the "triplication problem." Given a pentomino, use nine of the other pentominoes to construct a scale model that is three times as high and two times as wide as the given piece. Constructions of all twelve of the pentominoes are possible.

Not only do pentominoes have a wide appeal among mathematical-recreations fans, they also serve as fascinating "enrichment" material in school mathematics programs. There are at least five good reasons to incorporate pentominoes in the classroom:

1. - Pentominoes nurture a nonanxious attitude toward mathematics and science,
2. - promote an atmosphere of cooperation,
3. - support development of the problem-solving process,
4. - provide spatial-ability skill exercises,
5. - and introduce students to elementary number theory.

Through various studies, it has been discovered that students with anxieties toward mathematics and science perform less well on mathematics and science achievement tests. The informal geometry that is ingrained in pentomino discovery is an excellent nonthreatening activity with which to provide students. Pentominoes can also be used to examine the concepts of congruence, similarity, transformations (flips, turns, and slides), tessellations (tilting), perimeter, area, and volume in a relaxed atmosphere.

The value of cooperation can also be demonstrated through the use of pentominoes. The process of working together in small groups on pentomino problems promotes a desire among students to become involved in more formal cooperative learning activities in the future.

Pentomino activities provide good practice using the four-step problem solving process:

1. - understanding the problem
2. - devising a plan
3. - carrying out a plan
4. - checking the work

Skill in representing, transforming, generating, and recalling symbolic, nonlinguistic information is referred to as being spatial ability. Mental rotation, one type of spatial ability, requires students to rotate a two- or three-dimensional figure rapidly and accurately. Another type of spatial ability, spatial visualization, demands students to demonstrate an ability which involves complicated, multistep manipulations of spatially presented information. Spatial-ability skills have been found to correlate with mathematics and science achievement, and the ultimate goal of practicing spatial-ability skills is for students to be able to mentally manipulate figures.

Provoking questions can be asked about the family of -ominoes. The unique set of biominoes (shapes consisting of two squares joined together by at least one common side) can easily be discovered; there is only one unique biomino. The unique set of triominoes (shapes consisting of three squares joined together by at least one common side) can be quickly found; there are only two triominoes. Identifying the unique set of quadominoes (or tetrominoes) (shapes consisting of four squares joined together by at least one side) is also quite simple; there are five quadominoes. Even so, discovering the number of unique -ominoes is truly an exercise in analyzing number patterns. Although generating the number of unique -ominoes, uni through hex (1-6), is relatively simple, one should not be misled. Quite frequently, novice number theorists prematurely conclude that since a pattern holds for three or more cases, it will hold for an infinite number of cases; this is not true for the family of -ominoes. Even though computers have been programmed to semiconcretely generate the shapes of -ominoes, at this time, computers still are not able to be programmed to symbolically predict the number of unique -ominoes using a formula. The world of mathematics is anxiously awaiting its discovery.

Besides its intrigue as a puzzle, the placement of pentominoes on a checkerboard also make it an exciting competitive game of skill. Played by one, two, or three players, the object of the game is to be the last player to place a pentomino piece on the checkerboard. Players take turns choosing a piece and placing it on the board. The pieces must not overlap or extent beyond the boundary of the board, but they do not have to be adjacent. The game will last at least five, and at most twelve, moves, can never result in a draw, has more openings than chess, and will fascinate players of all ages. Although it is difficult to advise what strategy should be followed, there are two valuable strategic principles. First, try to move in such a way that there will be room for only an even number of pieces. (This applies only when there are two players.) Second, if a player is not able to analyze the situation, he should do something to complicate the placement so that the next player will have even more difficulty analyzing it than he did.

In conclusion, pentominoes provide intriguing puzzles, interesting patterns, and exciting games. They can also be used as effective teaching tools in the classroom. Pentomino activities nurture a nonanxious attitude towards mathematics and science, promote an atmosphere of cooperation, support the development of the problem-solving process, supply spatial-ability skill exercises, and introduce students to elementary number theory. Practicing such activities can give students positive perceptions about the world of mathematics and science. Furthermore, pentominoes furnish the means for initiating discussions about many mathematical concepts and serve as concrete representations that help ease students' understanding of abstract notions.